
|

|
Imprint • FAQs • Contact |
| Home |

|
|
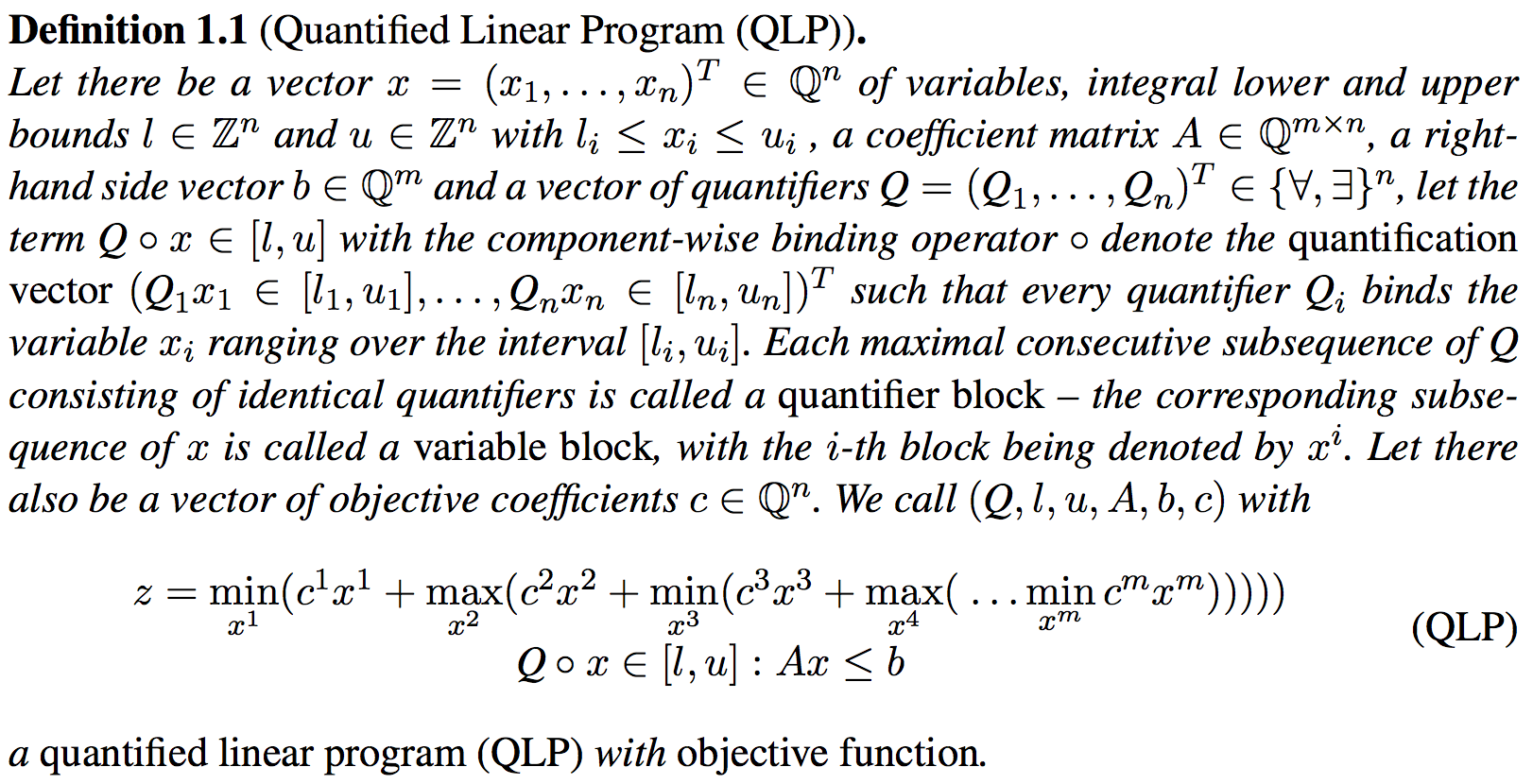
Quantified (Mixed) Integer Linear Programming
Mixed-integer linear programming (MIP) is a well-established
state-of-the-art technique for computer-aided optimization.
However, companies observe an increasing danger of disruptions
that prevent them from acting as planned. One reason is that
input data is often assumed to be deterministic, but in
reality, they are afflicted with uncertainties which cannot
be adequately described in MIPs, often. This is the point
where Q-MIPs are intended to support future modeling.
... as well as a restricted mixed version with the following
attributes:
[1] K. Subramani: On a decision procedure for quantified linear
programs. Ann. Math. Artif. Intell. 51(1): 55-77 (2007) |
Downloads
Yasol for Mac OS |
|
|
|
Imprint •
FAQs •
Contact
|